| Version 84 (modified by , 8 years ago) (diff) |
|---|
Project Name
| Infrastructure | CNRS_Coriolis |
| Project (long title) | Coriolis and Rotational effects on Stratified Turbulence |
| Campaign Title (name data folder) | 16CREST |
| Lead Author | Jeffrey Peakall |
| Contributor | Stephen Darby, Robert Michael Dorrell, Shahrzad Davarpanah Jazi, Gareth Mark Keevil, Jeffrey Peakall, Anna Wåhlin, Mathew Graeme Wells, Joel Sommeria, Samuel Viboud |
| Date Campaign Start | 12/09/2016 |
| Date Campaign End | 21/10/2016 |
1 - Objectives
Our primary objective is to measure detailed turbulence distributions within channelised gravity currents, as a function of Coriolis forces, concentrating on: i) the bottom boundary layer, ii) redistribution of turbulence within bends, and, iii) redistribution of turbulence at the interface between the gravity current and the ambient. These datasets will enable existing theory on the presence and influence of Ekman boundary layers to be tested, with important implication for the basal shear stress distributions, erosion, and the evolution of channels. These data on the distribution of turbulence will then be applied to i) examine the turbulence distribution in straight channels, ii) provide an analysis of secondary flow and associated turbulence around bends for the first time, and an assessment of how channelized flows alter as a function of Rossby numbers and therefore latitude, iii) assess how the morphodynamics of submarine channels vary as a function of the Rossby number, iv) explain the observed patterns of submarine channel sinuosity with latitude (Peakall et al., 2012; Cossu and Wells, 2013; Cossu et al., 2015), and, v) incorporate the entrainment data into numerical models of submarine channels, in order to address the unanswered question of how these flows traverse such large-distances across very low-angle slopes (Dorrell et al., 2014).Our primary objective is to measure detailed turbulence distributions within channelised gravity currents, as a function of Coriolis forces, concentrating on: i) the bottom boundary layer, ii) redistribution of turbulence within bends, and, iii) redistribution of turbulence at the interface between the gravity current and the ambient. These datasets will enable existing theory on the presence and influence of Ekman boundary layers to be tested, with important implication for the basal shear stress distributions, erosion, and the evolution of channels. These data on the distribution of turbulence will then be applied to i) examine the turbulence distribution in straight channels, ii) provide an analysis of secondary flow and associated turbulence around bends for the first time, and an assessment of how channelized flows alter as a function of Rossby numbers and therefore latitude, iii) assess how the morphodynamics of submarine channels vary as a function of the Rossby number, iv) explain the observed patterns of submarine channel sinuosity with latitude (Peakall et al., 2012; Cossu and Wells, 2013; Cossu et al., 2015), and, v) incorporate the entrainment data into numerical models of submarine channels, in order to address the unanswered question of how these flows traverse such large-distances across very low-angle slopes (Dorrell et al., 2014).
2 - Experimental setup:
2.1 General description
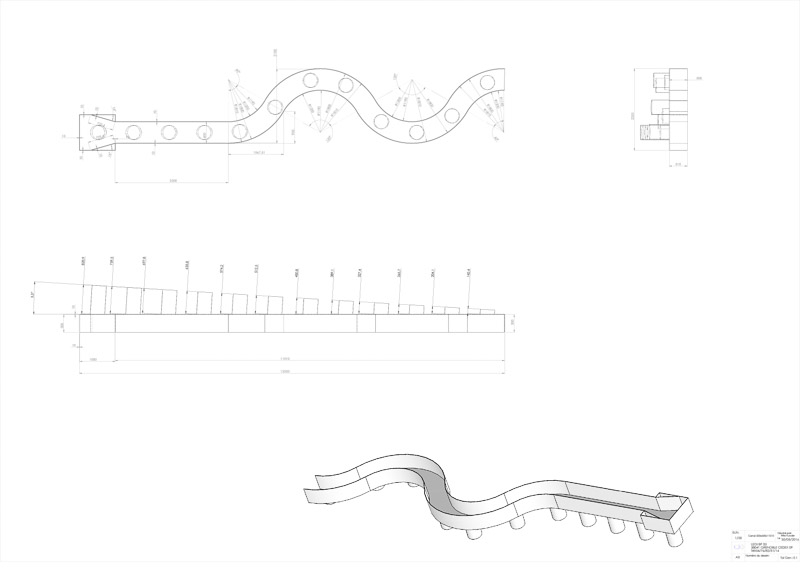
2.1 General description A channel model is positioned within the Coriolis facility. The channel model consists of an initial tapered input section with a honeycomb baffle for flow straightening and turbulence control, a 3.2 m straight channel section, and two bends with a mid-channel radius of 1.5 m. The channel is made of acrylic and is 60 cm wide and 50 cm high; the sinuous section has a sinuosity of 1.2. The slope is 3/50 radians (3.5 degrees, 6% gradient) and the channel terminates 10 cm off of the floor. Saline fluid is pumped into the top of the channel, forming a gravity current, which flows along the channel, and off the end. The basal 10 cm of the flume operates as a sump for the denser saline fluid to accumulate. In turn, this fluid can be drawn down in one of two ways: i) whilst recirculating the fluid, though this is limited to 20 $m3$/hr (5.55 l/s), and ii) through emptying to the drain, in which case any flow rate is possible. Two long metal rails are positioned to either side of the channel model across the full width of the flume. These carry a computerized gantry, which can be positioned at any point along the channel. The gantry itself contains the controls for two Schneider slides, one orientated transverse to the model, and the other connected slide, orientated in the vertical. Thus the system enables xyz control.
2.2 Definition of the co-ordinate system
2.3 Fixed Parameters
| Notation | Definition | Values | Remarks |
| $Q_0$ | Input Density | $12 \ ls-1$ | |
| $\Delta\rho$ | Density Difference | $20 \ kg \ m-3$ | |
| $W$ | Channel Width | $0.6 \ m$ | |
| $\nu$ | Viscosity | $10-6m2s-1$ | |
| $S$ | Slope | $3.5{\circ}$ |
2.4 Variable Parameters
| Notation | Definition | Unit | Initial Estimated Values | Remarks |
| $\Omega$ | Rotation Rate | $rads-1$ | -0.18 - 0.15 | |
| $H_w$ | Water Depth | $m$ | 1-1.1 | |
| $Q_o_u_t_p_u_t$ | Output Flow Rate | $ls-1$ | 5.5 - 17 | |
| $k$ | Roughness | - | - |
2.5 Additional Parameters
| Notation | Definition | Unit | Initial Estimated Values |
| $h$ | Depth of gravity current | $m$ | 0.1 |
| $U$ | Mean downslope velocity | $ms-1$ | 0.1-0.15 |
| $\delta$ | Thickness of Ekman boundary layer | $mm$ | ~10 |
| $R$ | Radius of curvature | $m$ | 1.5 |
2.6 Definition of the relevant non-dimensional numbers
Flow Reynolds number across the obstruction, $Re = Uh/\nu$.
Densimetric Froude number, $Fr = U/(g'h){1/2}$, $g' = g(\Delta\rho)/\rho_0$.
Rossby number, $Ro = U/fW$.
Canyon number, $\beta = sW/\delta$.
Bulk Richardson number, $Ri = 1/(Fr{2})$
Keulegan number, $Ke = (Ri/Re?){1/3}$
3 - Instrumentation and data acquisition -
3.1 Instruments
3.2 Definition of time origin and instrument synchronisation
6 - Table of Experiments:
| Run Name | Downstream (x)Position | Density Excess | Input flow | Rotation Rate | Initial Water Depth | Outflow Rate | Run Time | ADV Dwell Time |
| $(kg \ m-3)$ | $(ls-1)$ | $(rad \ s-1)$ | $(m)$ | $(ls-1)$ | $(Minutes)$ | $(s)$ | ||
| fixstr1_1909A | xx | 20 | 12 | 0 | 1 | 5.5 | 30 | continuous |
| fixstr1_2109A | xx | 20 | 12 | 0 | 1 | 5.5 | 20 | 60 |
| Smooth_3 | 2 | 20 | 12 | 0 | 1 | 5.5 | 20 | 60 |
| Smooth_4 | 3 | 20 | 12 | 0 | 1 | 5.5 | 20 | 60 |
| Smooth_5 | 4 | 20 | 12 | 0 | 1 | 5.5 | 20 | 60 |
| Smooth_6 | 1 | 20 | 12 | 0 | 1 | 5.5 | 20 | 30 |
| Smooth_7 | 3 | 20 | 12 | 0 | 1 | 5.5 | 20 | 30 |
| Smooth_8 | 4 | 20 | 12 | 0 | 1 | 5.5 | 20 | 30 |
| Smooth_9 | 2 | 20 | 12 | 0 | 1 | 12.0 | 60 | 30 |
7 - Diary:
Monday September 19th 2016
Experiment name: fixstr1_1909A Filenames: fixstr1_1909A1, fixstr1_1909A2 Location: Position 1 (75% of the way down the straight section, 58 cm upstream from the end of straight section). Input rate: 12 l/s, density excess: 20 kg/$m3$. Water was very cloudy to the extent that we were not able to use the laser. No siphon rig was used. Running basal ADV with ADV #1 located 7.2 cm from the base. ADV just measured at one point (no traverse measurement).
Experiment started at 2:45pm and stopped at 3:15 pm. The flow automatically stopped part way through as a valve was not open for recirculating water. Part way through red dye was added to visualise the current. Dye visualisation suggested pretty thin flow on the inner bank and significant super elevation on the outer bank. Some perturbation was observed on the surface of the flow at the outer bank, but otherwise the flow surface appears quite smooth, and mixing appeared to be very low. Mean maximum flow velocity from raw output was around 20 - 25 cm/s. ADV and UVP measured twice, the first is referred to as fixstr1_1909A1 and this had a velocity range of 0.3 m/s on the ADV setting, and 0.25 m/s for the UVP. Instantaneous flow velocities were faster than anticipated, as a result of the steep slope (3.5 degrees) with flow wrapping on both instruments so the velocity range was increased on the ADV and the UVP. A second run period fixstr1_1909A2 had a velocity range of 0.5 m/s on the ADV setting. The UVP setting was 680 mm/s (0.68 m/s).
Tuesday September 20th 2016
Paint was applied to the tank floor to address a leak in the flume, and the tank floor left to dry and seal. The siphon rig was completed and tested. The laser system was aligned. The position of the basal ADV was refined, Orgasol was required as additional seeding in order for bottom tracking to work effectively. Interesting, the stem (fixed) ADV picks this bottom point up better than the flexible ADV in the absence of seeding. Tested synchronization of ADV with traverse; a problem was identified with the nature of the required input signal. Investigation in progress as to how to address this.
Wednesday September 21st 2016
Attachments (5)
-
section 500x600 lg 11010.PDF (195.7 KB) - added by 8 years ago.
Drawing Model on Table
-
Set_up_drawing.png (212.8 KB) - added by 8 years ago.
Drawing
- Set_up_drawing.jpg (53.9 KB) - added by 8 years ago.
-
Traverse.pdf (46.1 KB) - added by 8 years ago.
Traverse set up for main experiments
-
CRESTTraverseMeasurement.jpg (340.5 KB) - added by 8 years ago.
CREST Traverse Measurement - main experiments
Download all attachments as: .zip